Prove that any chord [AB] of the larger circle is bisected by the smaller circle.

Question

Two circles touch at A. O is the center of the larger circle.  The smaller circle passesd through O.

Answer 1

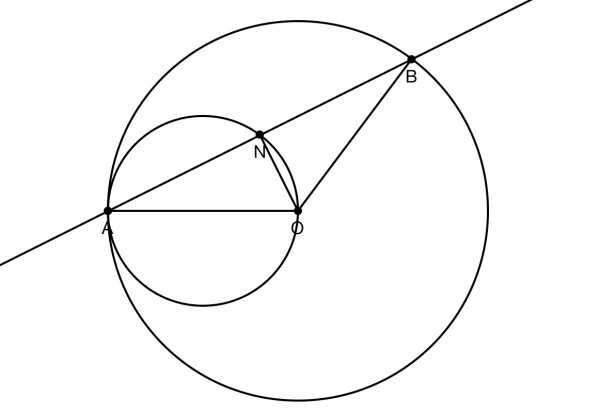
The small circle passes through O and touches the large circle at A, so AO is a radius of the large circle and a diameter of the small circle, since point A is a tangent point to both circles, and N is the intersection of the small circle with the large circle. Angle ANO is a right angle, because the angle in a semicircle is a right angle and the circle is the small circle. 

OB is a radius of the large circle. ON is perpendicular to AB. Angle ANO=ONB=90 degrees. ABO is an isosceles triangle because OA=OB=radius. ON is a common side, so triangles ANO and ONB are congruent (RHS-Right angle, Hypotenuse, Side). Therefore AN=NB so the small circle bisects the chord AB.