Find key points first. Intercepts are important. When is g(x)=0? The answer is: when x=4 and -1, so mark these points on the x-axis. Now, put x=0 into the function, that is, find g(0), to give you the y-intercepts: g(0)=-64. So mark -64 on the y-axis. Next, make out a small table with low values of x, say, x goes from -2 to 2. Work out corresponding values of g(x):
x g(x)
-2 216
-1 0 (already marked as an x-intercept)
0 -64
1 -54
2 -24
There’s no need to plot these points but you can use them to see how steep the graph is. However, if you extend the table to include values of x near 4 you might realise there’s a “kink” at x=4.
Here are two pictures of the graph with different scales.
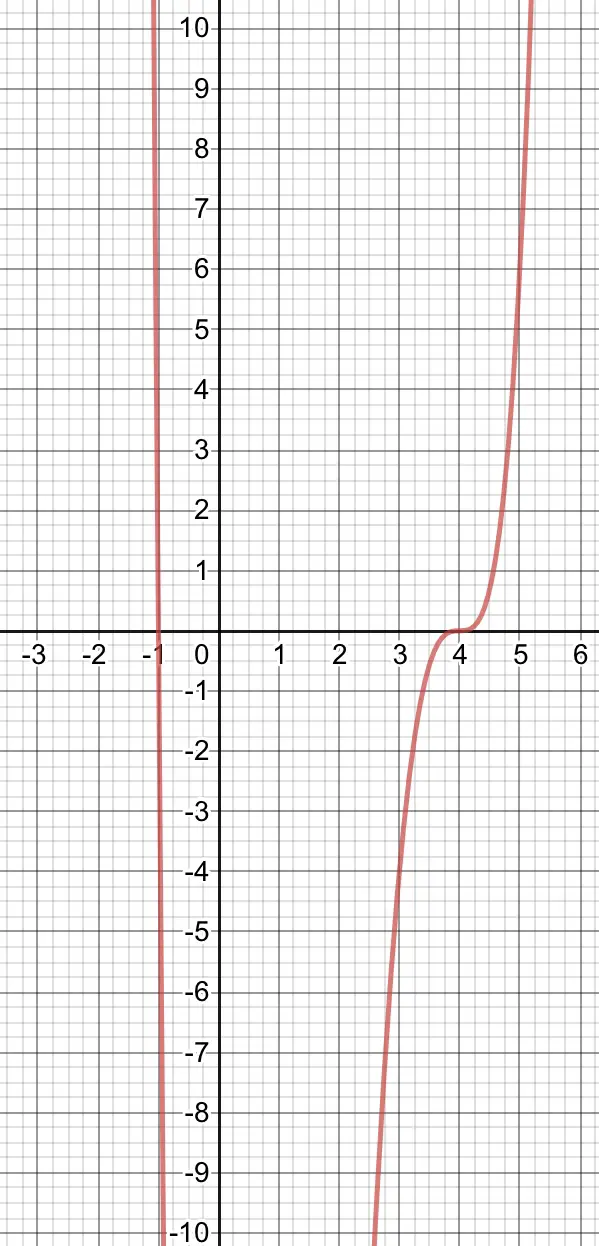
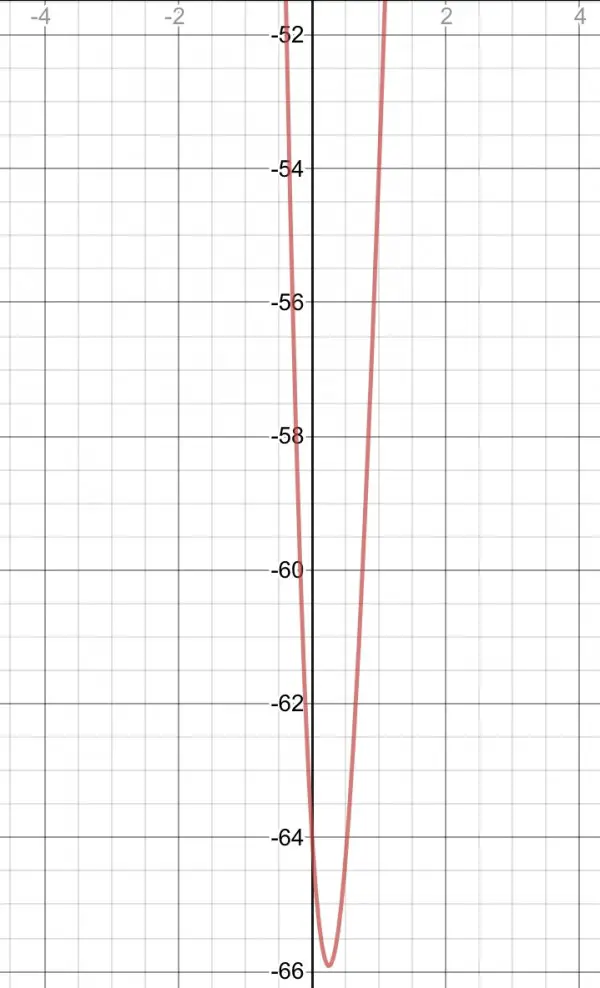
The lower graph shows the y-intercept and how the two parts of the graph shown in the upper graph join together near the y-intercept.