| The P |
Q |
¬P |
¬Q |
P⇒Q |
P⇔Q |
P∨Q |
P∧Q |
P∧¬Q |
¬P∧Q |
¬P∧¬Q |
¬(P∧¬Q) |
| T |
T |
F |
F |
T |
T |
T |
T |
F |
F |
F |
T |
| T |
F |
F |
T |
F |
F |
T |
F |
T |
F |
F |
F |
| F |
T |
T |
F |
T |
F |
T |
F |
F |
T |
F |
T |
| F |
F |
T |
T |
T |
T |
F |
F |
F |
F |
T |
T |
1. From the truth table above, when P and Q are both true, then P⇒Q (if P is true then Q is true), P⇔Q (P is true if and only if Q is true), P∨Q (either or both P or Q are true), so the three logical relationships are equivalent.
When P∧¬Q (P is true and Q is not true) is true, then we can replace Q in row 1, columns 5, 6, 7 with (P∧¬Q), e.g., P⇔(P∧¬Q), and similarly with the other two logical operations.
What we need to do is to use only ¬ and ∧ with P and Q to find equivalent logical expressions for each of the operations in columns 5, 6, 7. The table should help us to find them.
The last column, as an example, shows what is equivalent to P⇒Q. Here’s how I got the answer.
(I would have added more columns to include the answers I’ve written below, but the truth table would be too wide to read. I hope the explanations below help you to understand the processes.)
P⇒Q gives us the column T F T T. Looking across the table I saw that column P∧¬Q was F T F F which is the complement of what I needed. The NOT operator (¬) converts F to T and T to F, so by writing ¬ in front of P∧¬Q I would get the result I needed. This is written ¬(P∧¬Q).
P⇔Q is a bit trickier, but I got the answer ¬(P∧¬Q)∧¬(¬P∧Q).
P∨Q is ¬(¬P∧¬Q).
2.
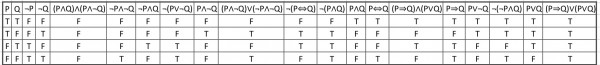
This is what the question is looking for. There are other solutions, so other column headers are possible. To show you how to do this, let’s take an example. Take column 15, TFTF. In the columns with a header already, we look for another column which already has a header and which looks similar to TFTF. I used the next column 16, P⇒Q: TFTT. All I need is something to change the last T into an F. I also need a logical operation to turn T into F. The operation is AND (∧). T∧F=F. So I noticed that column 19 contained an F and the rest were T’s so combining columns 16 and 19 gave me the result I needed: TFTT ∧ TTTF=TFTF. This gave me (P⇒Q)∧(P∨Q) for column 15’s header. This method turns the problem into a game.
Continued in comment...