Given ABC, BD bisects angle B, AD = 8, AB = 12, BC = 18. Find DC.

Question

There is no model and I am vey confused on where to start, if you culd also show work on how you got the answer it would be extremely helpful. Thank You!

Answer 1

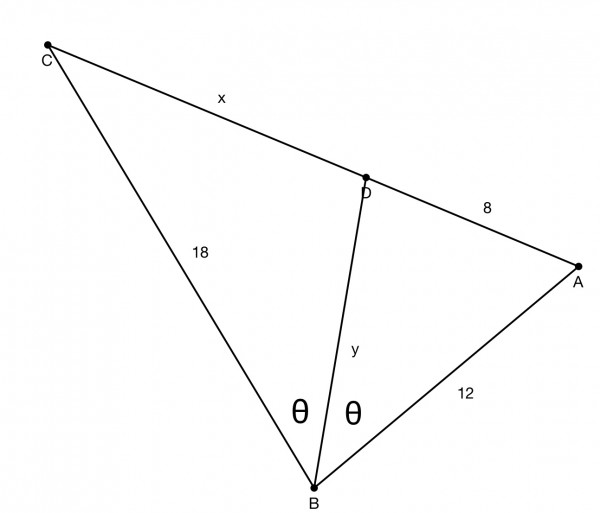
The angle bisector splits triangle ABC into two triangles ADB and DBC. The sum of the areas of the two triangles equals the area of ABC. We use the formula ½bcsinA where A is the angle between two sides. So we can write ½AB.BDsinθ+½BC.BDsinθ=½AB.BCsin(2θ) where 2θ is the measure of angle B. Let x=DC and y=BD and substitute AB=12 and BC=18:

6ysinθ+9ysinθ=108sin(2θ)=216sinθcosθ.

sinθ can be cancelled out: 15y=216cosθ and cosθ=15y/216=5y/72. Whenever we encounter cosθ in other equations we can replace it with 5y/72.

In triangle ABD we can apply the Cosine Rule.

8²=y²+12²-24ycosθ=y²+144-24y(5y/72).

So: 64=y²+144-5y²/3. ⅔y²=80, y²=120.

In triangle DBC we can apply the Cosine Rule.

x²=y²+18²-36ycosθ=y²+324-36y(5y/72).

So: x²=y²+324-5y²/2=324-3y²/2=324-360/2=144, and x=12.

x=DC=12.