Question

A villager walks 6km on a bearing of 37° and than walks a further 7km on a bearing of 320°. At this point this point he stops to rest . Draw a scale drawing of the villagers journey using a scale of 1:10000 B: Use the cosine rule to calculate the distance of the villagers resting point from his starting point. C: Measure the distance the villagers resting point and his starting point and convert this to kilometers. D: Find the bearing of the starting point from the resting point , to the nearest degree.

Answer 1

On this scale 1/10000km on the drawing is equivalent to 1km on the walk.

1km=1000m; 1m=100cm, so 1km=100000cm. 100000/10000=10cm.

Therefore 10cm on your drawing is the equivalent of 1km.

0.1km=100m is represented by 1cm. So you can write the scale as 1cm=100m.

6km≣60cm on the drawing, 7km≡70cm on the drawing (that’s a large drawing!).

Here’s the shape drawn to scale:

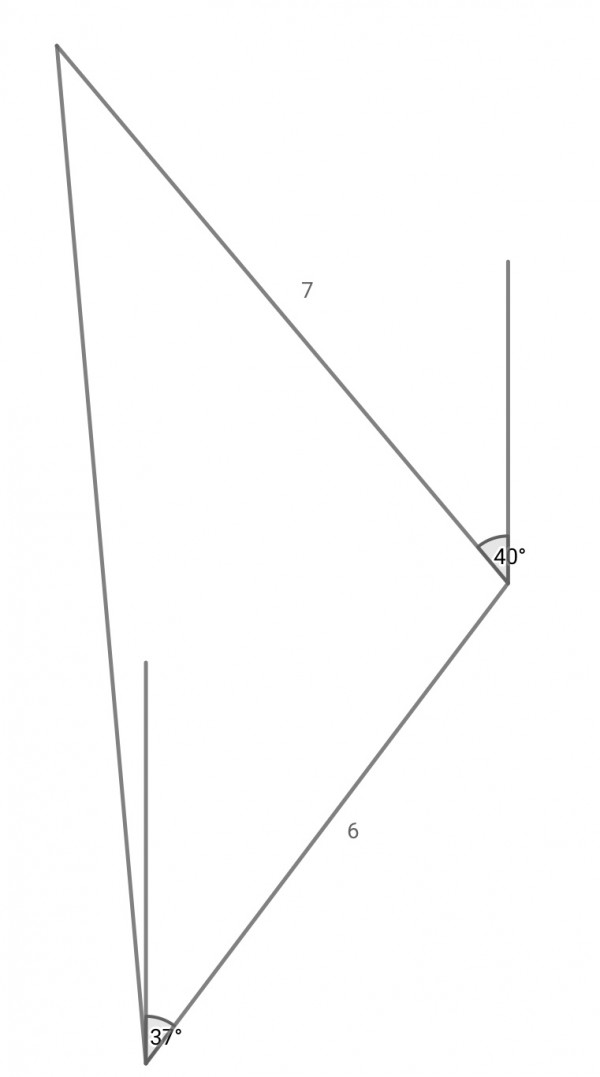
I’ve taken the bearing angle to be measured from north clockwise. The angle 40° is 360-320=40° anti-clockwise from north.

Cosine Rule gives us the distance from the starting point to the resting point, x:

x²=6²+7²-2×6×7cos(180-(40+37))=85-84cos103=103.896 approx. Therefore x=10.193km, which will be 101.93 cm  on the scale drawing. You will probably measure this as 101.9 or 102 cm on your drawing.

The Sine Rule can be used to find the bearing of the resting point from the starting point:

Start with x/sin103=7/sinθ. The bearing will be derived from angle θ.

From this sinθ=7sin103/x=0.66915 approx. So θ=42.001° approx. Subtract 37° and we get the anti-clockwise bearing=5.001°. The clockwise bearing is 360-5.001=355° to the nearest degree.