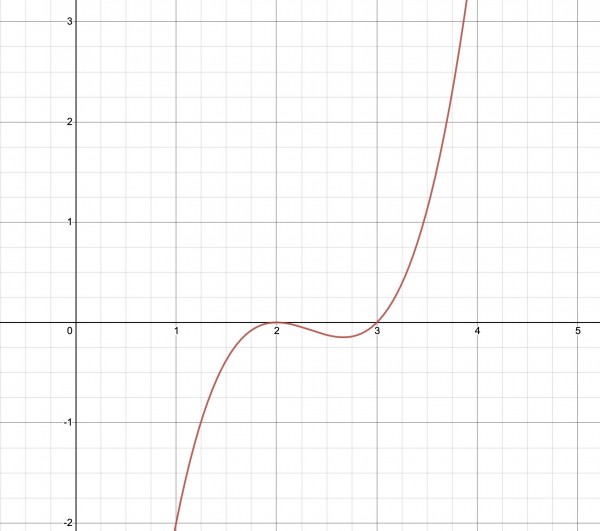
Here are some clues. When x=2 and 3, y=(2-x)2(x-3) is zero, so mark the x-intercepts at (2,0) and (3,0). When x=0, the y-intercept is (0,-12), so mark this point. When x is a little less or more than 2, y is negative so the graph rises from (0,-12) to touch the x-axis at x=2 before descending a little, then ascending to cut the x-axis at x=3 and then continuing to rise as x increases. So there is a maximum at (2,0) and a minimum between x=2 and 3. When x=2.5, for example, y=-⅛.