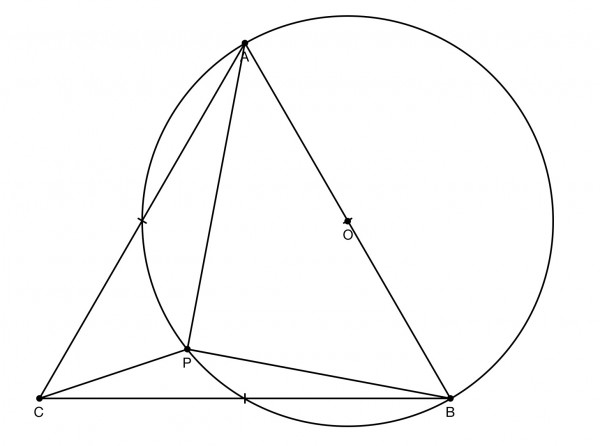
If AP^2=BP^2+AB^2, then angle B is a right angle. But that would put P outside the triangle.
Also, since BP is not zero length then AP must be longer than AB, which is the length of each side of the triangle, a. P cannot be inside the triangle because there is no line longer than the side of the triangle which is also inside the triangle. The greatest distance from A is a (AB or AC).
If P is outside the triangle then as long as B is a right angle, angle BPC can have any size less than 150 degrees, because CPB=90-ABC=90-60=30 degrees and BPC+BCP=180-CBP=180-(90-ABC)=180-(90-60)=180-30=150.
But if AB^2=AP^2+PB^2 then P is inside the triangle.
If AB is the diameter of a circle then the midpoint of AB is O the centre of the circle. Draw a circle with radius AO. It will pass through vertices A and B. P can be any point on the circle inside the triangle. Because P can move about on the circle there is no fixed angle for BPC.